Fundamentals of Spatial Filtering
Fundamentals of Spatial Filtering
Fundamentals of Spatial Filtering
Spatial filters provide a convenient way to remove random fluctuations from the intensity profile of a laser beam, which can be critical for applications like holography and optical data processing.
Laser beams pick up intensity variations from scattering by optical defects and particles in the air. This is easily visualized by expanding a laser beam onto a screen where the patterns, holes, and rings superimposed on the ideal pattern of uniform speckle are due to scattering.
Spatial filtering is conceptually simple (see Figure 1). An ideal coherent, collimated laser beam behaves as if generated by a distant point source. Spatial filtering involves focusing the beam and producing an image of the "source" with all its scattering imperfections defocused in an annulus about the axis.

The ideal Gaussian laser beam profile, I(r), is contaminated by intensity fluctuations, δΙ, caused by scattering. δΙ varies rapidly over an average distance dn, which is much smaller than the beam radius, a. The distance dn is then known as the average spatial wavelength of the laser beam noise (see Figure 2).
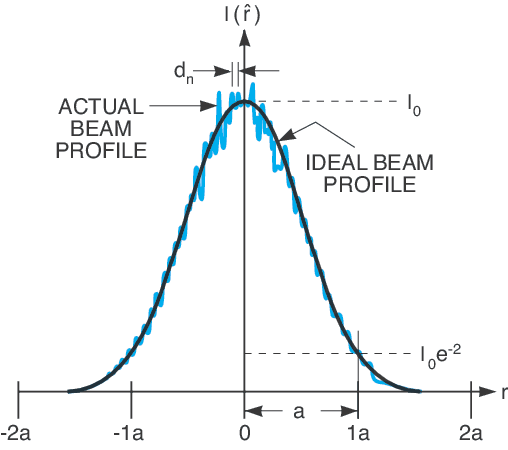
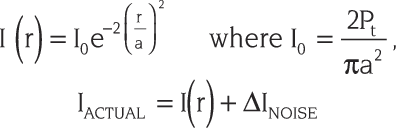

When a positive lens of focal length F focuses a Gaussian beam, the image at the focal plane (the Optical Power Spectrum, or OPS) will be an inverted “map” of spatial wavelengths present in the beam. Short wavelength noise (dn) will appear in an annulus of radius Fλ/dn centered on the optic axis. The long spatial wavelength of an ideal Gaussian profile will form an image directly on the optic axis (see Figure 3).
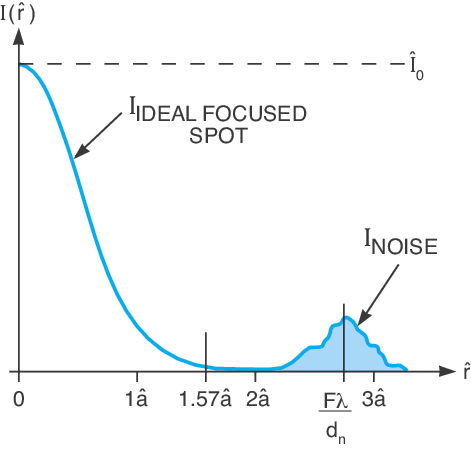
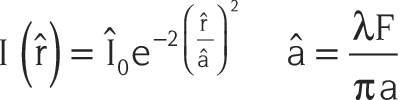

A pinhole centered on the axis can block the unwanted noise annulus while passing most of the laser's energy. The proper pinhole size depends on the focal length of the focusing lens or objective and the diameter of the original beam. It is necessary to make certain that the energy density of the beam at the focal point does not exceed the damage threshold limitation of the pinhole material. A spatial filter can be set up on an optical rail system. However, compact spatial filter assemblies are also typically available. Proper positioning of the pinhole with respect to the focused beam is made using a positioner that allows for three degrees of translation.
The fraction of power passed by a pinhole of diameter D is:
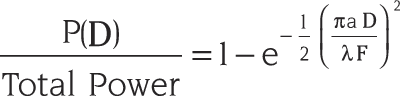
and the minimum noise wavelength transmitted by the pinhole is
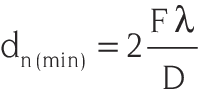
The optimal pinhole diameter Dopt is:
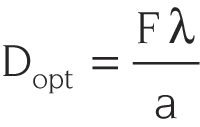
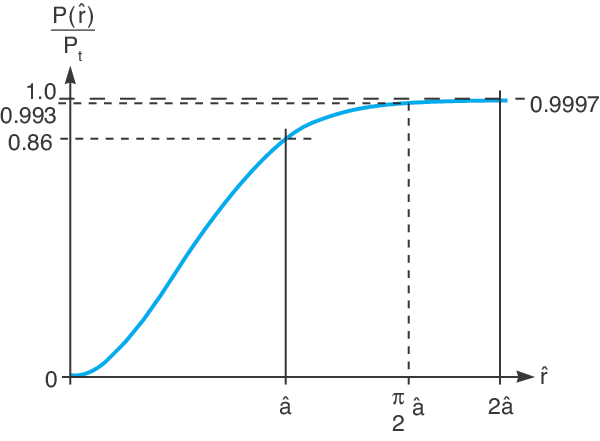
This passes 99.3% of the total beam energy and blocks spatial wavelengths smaller than 2a, the diameter of the initial beam. Since dn is always much smaller than the beam diameter, the filtered beam is very close to the ideal profile (see Figure 4)

Related Topics
Optical Components
- Optical Mirror Physics
- Optical Lens Physics
- Diffraction Grating Physics
- Optical Coatings
- Optical Filters
- Polarization Optics
Optical Systems